Na Avogadro
Mole, Polarity, Intermolecular Forces, Avogadro's Number
Avogadro's Constant is not dimensionless - which is the big difference between the two. The Constant has units of reciprocal quantity. The Constant has units of reciprocal quantity. If that quantity is reciprocal moles, then the numerical value of the Constant and the Number are identical, but the quantity could be something else, for instance. Example Exercise 9.1 Atomic Mass and Avogadro’s Number. The atomic mass of each element is listed below the symbol of the element in the periodic table: Cu = 63.55 amu, Hg = 200.59 amu, S = 32.07 amu, and He = 4.00 amu. The mass of Avogadro’s number of atoms is the atomic mass expressed in grams. Therefore, 6.02. Avogadro’s number NA is 6.02 x 1023 mol-1, which is the same as 6.02 x 1023 atoms or molecules per mole. It is based on a theory by Italian physicist Amedeo Avogadro (1776-1856). He had learned about Gay-Lussac’s Law, and conjectured that all gases with the same temperature and pressure at the same volume also have the same number of. The number of carbon atoms in exactly 12 g is called Avogadro's number,.One mole is the amount of material which contains Avogradro's number of particle. These definitions emphasize that the mole refers to a fixed number of b Avogadro's number of atoms, electrons to refer to a mole of helium, a mole of electrons, or a mole of by Avogadro's number of atoms, electrons or ions respectively.
To estimate the number of stearic acid molecules in a mole of stearic acid based on an assumption about a thin film of stearic acid on a water surface. A monolayer of stearic acid can be created in which we can estimate the number of molecules. To do this we make assumptions of the size and shape of the stearic acid molecule.
The number you calculate for your conclusion will not match Avogadro's number to four significant figures, but should be within a power of 10 of the accepted value. This process should help you get a feel for the size of a mole and help you understand the value of counting molecules by moles rather than by singles.
In the International System (SI) of units, the fundamental measure of quantity is the mole. A mole is 6.022 x 1023 singles, just like a dozen is 12 singles. A dozen is an exact number because a dozen is defined to be 12 singles. A mole is defined differently. A mole is defined as the number of carbon-12 atoms in 12 grams of carbon-12. To six significant figures this measures 6.02214 x 1023 single atoms. To four significant figures, this measures 6.022 x 1023 single atoms.
A mole is useful for the same reason a dozen is useful. When you're baking donuts, it's hard to keep track of individual donuts and it's more useful for a baker to know how many dozen donuts he has than to know how many single donuts are on his shelves. The same is true of a chemist. On a laboratory scale, it's not practical to worry about how many gold atoms or caffeine molecules are in a beaker. It's more useful to know if you have 2.5 moles of gold atoms or 12.7 moles of caffeine molecules in your beaker.
The number of singles in a mole, 6.022 x 1023, is called Avogadro's number (NA). For this class, unless stated otherwise you should use Avogadro's number to four significant figures.
In this experiment you will estimate Avogadro’s number by calculating the amount of stearic acid necessary to form a single layer on the surface of water. This single layer is called a monolayer. We will be making some assumptions about how stearic acid molecules arrange themselves to form the monolayer. From these assumptions we can determine the thickness of the layer, and from the thickness we can estimate the number of the stearic acid molecules. If we know the volume occupied by a mole of stearic acid molecules we can use the volume of one stearic acid molecule to estimate Avogadro’s number. We simply divide the volume of a mole of stearic acid by the volume of one stearic acid molecule.

One of the properties displayed by water is its polarity. For covalent polar molecules, it is a partial charge separation denoted by a delta, δ. These polar ends are referred to as dipole moments. Polar molecules attract each other in much the same way magnets do. The negative end of the dipole moment of one polar molecule is attracted to the positive end of the dipole moment of another polar molecule. Because of this, acetic acid, CH3COOH, will dissolve in water. Whereas, pentane, CH3CH2CH2CH2CH3, a non-polar molecule is not water soluble. It stands to reason that a molecule that possesses both a nonpolar and polar end will have the polar end attracted to water and the nonpolar end be repelled by water.
Stearic acid, CH3(CH2)16CO2H, has the structure shown below. The acid has a polar portion and a non-polar portion. The large non-polar, hydrophobic portion, of the molecule makes it immiscible with water.
We will create a layer of stearic acid one molecule thick on the surface of a watch glass filled with water. The polar head group will stay near the water and the non-polar tail will orient themselves away from the water.
We will create our monolayer by using a solution of the stearic acid in hexane. Hexane is also hydrophobic and will not mix with water. The hexane will evaporate leaving just the thin layer of stearic acid.
If we know the area of the monolayer and we know the volume of the monolayer, we can measure the thickness, t, of the monolayer. This thickness will correspond to the length of the stearic acid molecule. If we make the assumption that the stearic acid molecule is a rectangular solid, with a width and length equal to 1/5.44 of the height, where the height equals the thickness of the monolayer, we can calculate the volume of one stearic acid molecule. Knowing the volume of the monolayer and the volume of one molecule we can calculate the number of molecules in the monolayer. By using the density of stearic acid we can determine the number of moles in the monolayer. Finally, we can use the molar volume of stearic acid and divide by the volume of the stearic acid molecule to get Avogadro’s number, NA!
There are two parts to this experiment. In part 1, we will calibrate a dropping pipet so that we can determine the number of drops in 1 mL. This will require a Pasteur pipet for optimum results. In part 2, we will slowly add the stearic acid/hexane solution to a watch glass of water. We will allow the hexane to evaporate leaving just the stearic acid on the water.
Any impurities will ruin this experiment so be very careful not to put soap, water or dust where it does not belong. Do not use dirty glassware!
Watch Glass
10 mL Beaker
10 mL Graduated Cylinder
Pasteur Pipet
Ruler (metric)
Stearic Acid
Hexane
Aqueous Ammonia Solution (Ammonium Hydroxide) - may be labeled NH3 (aq) or NH4OH
ALL WASTE HEXANE MUST BE DISPOSED OF INTO THE ORGANIC WASTE CONTAINERS. DO NOT DUMP HEXANE IN THE SINK.
Part 1: Calibration of the Pipet
- Obtain a Pasteur pipet from your instructor
- Obtain a 10 mL beaker from the stock room. Wash it well. Wash the inside 3 times with approximately 1 mL of aqueous ammonia. Rinse the beaker 3 times with distilled water. Rinse the beaker 1 time with acetone and then dry the beaker thoroughly with a paper towel. Rinse the beaker three times with approximately 1/2-mL of hexane and then add 3 mL of hexane to the beaker.
- Obtain a clean dry 10 mL graduated cylinder from your drawer. If your graduated cylinder is not clean and dry, clean it and then dry it with acetone and a tissue. Add hexane drop-wise to a volume of 1 mL. Record the number of drops.
- Repeat this procedure.
- If the two values are relatively close (within 10% of each other), then find the average. If not, repeat step 3 again.
- Dispose of excess hexane in the appropriate waste container and save the beaker for part 2.
Part 2: The Monolayer
- Obtain a watch glass from your drawer. Measure the diameter. Thoroughly clean the watch glass by scrubbing with soap for 2 minutes. Rinse well with tap water and then scrub with aqueous ammonia. Rinse 3 times with tap water and then three times with distilled water. Hold the glass by the edges to avoid putting fingerprints on it.
- Place the watch glass on a beaker; making sure that the watch glass is parallel to the floor. Fill the watch glass to the brim with distilled water.
- Take your clean 10 mL beaker and pour into it the stearic acid/hexane solution from under the hood.
- Draw some of the stearic acid/hexane solution into your Pasteur pipet, hold the dropper perpendicular to the surface of the water in the watch glass, and add one drop of the stearic acid solution.
- The solution should spread out rapidly across the surface of the water and disappear within a few seconds.
- If the watch glass is not properly cleaned then an oily residue may appear after only a few drops of solution. In this case it will be necessary to clean the watch glass again.
- If the first few drops disappear rapidly, continue adding the solution drop-wise, counting the drops.
- As the monolayer nears completion, the drop of solution forms a circular pattern rather than flowering out.
- The circular film of solution contracts as it evaporates and disappears in a relatively short time.
- This pattern will be observed for a few drops until finally, one drop strikes the surface and remains as a lens or globule that requires a prolonged period of time to disappear.
- Record the number of drops. At this point, the surface of the water is covered with a monolayer of stearic acid and one more drop placed at a different point on the water surface forms a second “lens”.
- Fill out the following table. You must show your calculations for all starred rows. Clearly show these calculations using dimensional analysis.
The following data must be collected during your experiment. Organize your data in a table similar to the layout shown below when you present data in your final report.
Pure Stearic Acid | Stearic Acid in Hexane Mixture | Pure Stearic Acid |
|---|---|---|
( 0.870 g = 1 mL ) | ( 284 g = 1 mol ) |
| Drops of Pure Hexane in 1 mL |
| Diameter of water surface (cm) | ||
| Drops of Hexane & Stearic Acid Mixture |
C1. Calculate the mass of stearic acid in the monolayer.
C2. Calculate the volume of stearic acid in the monolayer in mL
C3. Calculate the thickness of the monolayer in cm.
C4. Calculate the volume of a stearic acid molecule in the monolayer.
C5. Calculate the number of stearic acid molecules in the surface layer.
C6. Calculate the number of moles of stearic acid in the surface layer.

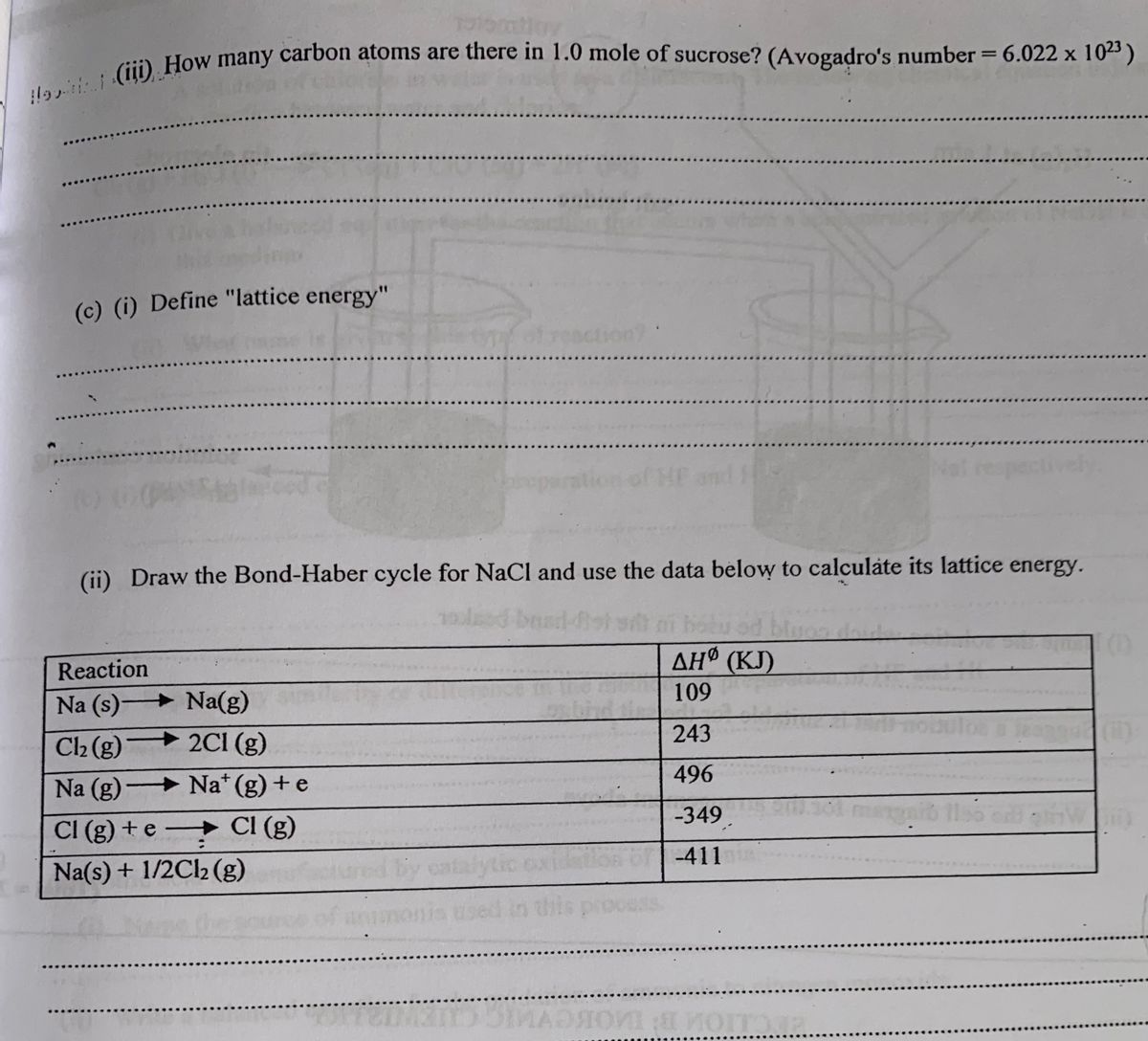
C7. Calculate the value of Avogadro’s number.
Alternate data (use only if your instructor indicates):
- Drops of hexane/mL 75 drops
- Number of drops hexane used for monolayer 27 drops
- Diameter of water surface 5.20 cm
Na Numero De Avogadro
Prepare a lab report about your experiment. Your lab report should contain the following sections. Each section should start a new page, with the section title clearly written at the top of that page.
- COVER SHEET
- DATA
- CALCULATIONS/REASONING
- CONCLUSIONS
- QUESTIONS
The standard cover sheet is available on the class website. Download this sheet as a PDF. Print and fill out this sheet and attach it as the first page of your report.
The DATA section of your report must contain all observations indicated in the data section of the lab description. Format data as a table whenever possible. If the experiment has multiple parts, divide your data section into those parts and clearly indicate which part of the experiment each data table belongs to.
Your interpretation of that data should be provided in CALCULATIONS/REASONING section. This includes the reasoning for all conclusions as well as any essential calculations
Na Avogadrov Broj
Respond to all objectives of the experiment in your CONCLUSIONS section. Clearly and concisely present your final answers here.
Q1. What is the mass of 5.47 moles of stearic acid? (strearic acid weighs 284 g per mole)
Q2. The density of stearic acid is 0.870g/mL. What is the volume of 7.8 x 10-6 mole of stearic acid?
Na Avogadro Konstante
Q3. One mole of iron atoms weighs 55.85 grams. If you have a 2.56 gram iron bar, how many single iron atoms are there in it? How many moles of iron atoms are in that same bar?
Q4. What is the mass, in grams, of 5 molecules of stearic acid?
Molecules and atoms are extremely small objects - both in size and mass. Consequently, working with them in the laboratory requires a large collection of them. How large does this collection need to be? A standard needs to be introduced. This standard is the 'mole'. The mole is based upon the carbon-12 isotope. We ask the following question: How many carbon-12 atoms are needed to have a mass of exactly 12 g. That number is NA - Avogadro's number. Thus, NA is defined by Careful measurements yield a value for NA = 6.0221367x10^+23. This is an incredibly large number - almost a trillion trillion. For example, if we stack NA pennies on top of one another how tall would the stack be? The answer is it would be so tall that the stack of pennies could reach the sun and back almost 500 million times! A convenient name is given when there is an Avogadro's number of objects - it is called a 'mole'. Thus in the above example there was a mole of pennies. The mole concept is no more complicated than the more familiar concept of a dozen : 1 dozen = 12 objects. From the penny example above one might suspect that the mass of a mole of objects is huge. Well, that is true if we're considering a mole of pennies, however a mole of atoms or molecules is a different story. Recall that the atomic mass unit (amu) is defined as 1/12 the mass of a carbon-12 atom. Consequently we have the relation Thus, a mole of carbon-12 atoms has a mass of just 12 g. What about other atoms? In the periodic table the atomic mass of the elements is given. For example the atomic mass of magnesium is 24.305 amu. This is the average isotopic mass of naturally occurring magnesium. What is the molar mass of magnesium in grams? From the equation above we get 1 amu = 1g/NA or 1 amu = 1.66054x10^-24 g. Thus, a mole of magnesium atoms has a mass of NA x 24.305 amu x (1.66054x10^-24 g/amu) = 24.305 g. A mole of magnesium atoms has a mass of 24.305 g. This example demonstrates that the atomic mass of magnesium can be interpreted in one of two ways: (1) the average mass of a single magnesium atom is 24.305 amu or (2) the average mass of a mole of magnesium atoms is 24.305 g; A similar conclusion follows for all of the other elements. |
Na Bilangan Avogadro
Back to index
Na Avogadro
